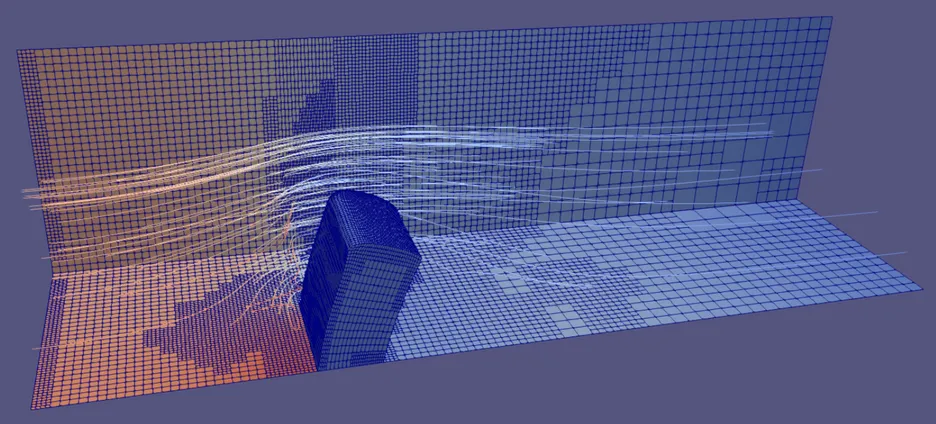
At the Chair of Optimal Control, we analyze and solve optimization problems, that are governed by partial differential equations. Problems of this kind arise in many different fields of application, examples being engineering, medicine or environmental science. Their variety ranges from optimal design problems, like shape or topology optimization, over optimally controlling time dependent processes like flow or cooling/heating problems, to parameter estimation problems from measured data. Often, additional constraints are imposed on the control or on the solution variables of the differential equation system.

In our research group, we formulate and analyse optimal control problems on a functional analytic level, i.e., as infinite dimensional problems. To solve them numerically, we develop specially tailored algorithms that combine state of the art optimization methods with modern adaptive techniques for discretizing the governing differential equations. The derivation of a priori and a posteriori error estimates for the discretized differential equations and the whole optimal control problems complements our development of new algorithms.

The research at the Chair of Optimal Control currently focuses on:

• Optimal Control of time dependent PDEs
• Boundary Control
• Measure Valued Control
• Machine Learning
• Flow Control